Are you curious about how far A Body In Free Fall Can Travel while exploring the beautiful landscapes of Vietnam? At SIXT.VN, we provide insights into the physics of free fall and offer services to enhance your travel experience. Discover convenient solutions for your Vietnam trip with reliable booking services, airport transfers, and customizable tour packages.
1. What Is Free Fall And How Does It Apply To Travel In Vietnam?
Free fall refers to the motion of an object solely under the influence of gravity. In the context of traveling in Vietnam, while you won’t experience true free fall in the literal sense, understanding the concept helps appreciate the physics involved in activities like ziplining or even the descent from mountainous regions.
Free fall is defined as the state where an object is moving exclusively under the influence of gravity, with no other forces acting upon it, such as air resistance. This concept, fundamental in physics, helps us understand how objects accelerate towards the Earth.
Applying Free Fall Concepts to Travel Experiences
While actual free fall might not be part of your typical Vietnam itinerary, understanding its principles can enhance your appreciation of various adventure activities:
-
Ziplining: Experiencing the thrill of ziplining involves a descent that, while not pure free fall, is influenced by gravity. The initial drop and subsequent glide are governed by gravitational acceleration, modified by the zipline’s tension and air resistance.
-
Mountain Descents: Hiking or trekking down Vietnam’s stunning mountains, such as those in Sapa or Ha Giang, means managing descents where gravity plays a significant role. Understanding how gravity affects your movement can help you control your pace and prevent accidents.
Factors Affecting Real-World Fall Scenarios
In real-world scenarios, several factors complicate the pure physics of free fall:
-
Air Resistance: Unlike a vacuum where free fall is perfect, air resistance significantly impacts how objects fall on Earth. This force opposes the motion of an object through the air, reducing its acceleration.
-
Object Shape and Size: The shape and size of an object influence the amount of air resistance it encounters. A larger surface area experiences more resistance, slowing the fall.
-
Altitude: Gravitational acceleration slightly varies with altitude. At higher elevations, the force of gravity is marginally weaker, affecting the rate of descent.
By understanding these factors, travelers can better appreciate the physics behind various activities and how external elements influence their experiences. Whether you’re considering the dynamics of ziplining or the caution required while descending a steep mountain trail, knowing the basics of free fall can add depth to your adventures in Vietnam.
2. What Factors Affect How Far A Body In Free Fall Can Travel?
Several factors influence the distance a body in free fall can travel, including time, initial velocity, and air resistance. In Vietnam, these factors are particularly relevant when considering activities involving height and descent, such as exploring caves or waterfalls.
The distance a body travels in free fall is determined by a few critical factors, primarily time, initial velocity, and air resistance. Here’s a more detailed look at each:
Time
The duration of the fall is the most straightforward factor. The longer an object falls, the greater the distance it covers. The relationship between time and distance in free fall is quadratic, meaning the distance increases exponentially with time.
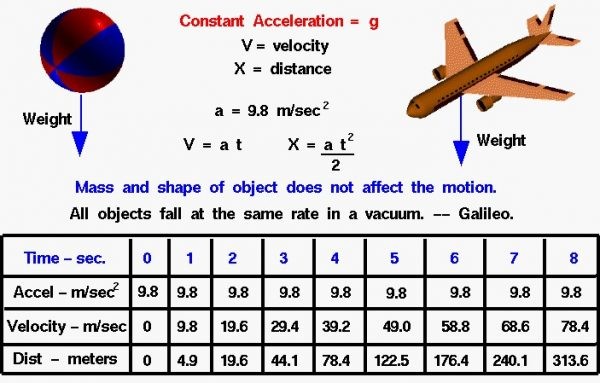
- Formula: The basic equation to calculate the distance (d) an object falls in a vacuum is ( d = frac{1}{2}gt^2 ), where ( g ) is the acceleration due to gravity (approximately 9.8 m/s²) and ( t ) is the time in seconds.
Initial Velocity
An object may have an initial velocity (speed and direction) before it starts to fall. If the object is simply dropped, its initial velocity is zero. However, if it’s thrown downward, the initial velocity adds to the distance covered during the fall.
- Impact: The total distance fallen will be greater if the object starts with a downward velocity. The equation becomes ( d = v_0t + frac{1}{2}gt^2 ), where ( v_0 ) is the initial velocity.
Air Resistance
Air resistance is a force that opposes the motion of an object through the air and significantly affects free fall, especially over longer distances. It depends on several factors:
-
Object’s Shape and Size: A larger object with a greater surface area encounters more air resistance. Aerodynamic shapes experience less resistance than irregular ones.
-
Velocity: Air resistance increases with the square of the velocity. As an object falls faster, the air resistance force becomes stronger, eventually reaching a point where it equals the gravitational force.
-
Air Density: Air density varies with altitude and temperature. Higher altitudes have lower air density, resulting in less air resistance compared to lower altitudes.
Terminal Velocity
Due to air resistance, an object in free fall eventually reaches a constant speed called terminal velocity. At this point, the force of air resistance equals the force of gravity, and the object stops accelerating.
-
Definition: Terminal velocity is the maximum speed an object can reach during free fall in an atmosphere.
-
Factors: The terminal velocity depends on the object’s weight, shape, and the density of the air. For example, a skydiver with an open parachute has a much lower terminal velocity than one without.
Understanding these factors is crucial for predicting and analyzing the motion of objects in free fall. Whether you’re a physicist, engineer, or simply curious about the world around you, grasping these concepts provides valuable insights into the behavior of falling objects.
 Cave exploration in Vietnam
Cave exploration in Vietnam
Practical Implications in Vietnam
-
Cave Exploration: When exploring caves like Son Doong, understanding these factors can help estimate the depth and potential hazards of underground spaces.
-
Waterfall Safety: Considering the height and force of water at waterfalls like Ban Gioc can enhance safety measures.
By understanding these principles, travelers can better appreciate the forces at play in Vietnam’s natural environments and make informed decisions about their safety and enjoyment.
3. How Is Acceleration Related To Free Fall Distance?
Acceleration, particularly the acceleration due to gravity, is directly related to the distance an object travels in free fall. This relationship is described by kinematic equations, which are essential for understanding and predicting the motion of falling objects.
Acceleration is a fundamental concept in physics that describes the rate at which an object’s velocity changes over time. In the context of free fall, acceleration is primarily due to gravity, which exerts a constant downward force on objects near the Earth’s surface. Understanding the relationship between acceleration and free fall distance is crucial for analyzing the motion of falling objects.
Acceleration Due to Gravity
-
Definition: The acceleration due to gravity, often denoted as ( g ), is the constant acceleration experienced by objects in free fall near the Earth’s surface.
-
Value: The standard value of ( g ) is approximately 9.8 meters per second squared (9.8 m/s²), which means that an object’s downward velocity increases by 9.8 meters per second every second it falls.
Kinematic Equations
Kinematic equations are a set of formulas that describe the motion of objects under constant acceleration. These equations relate displacement (distance), initial velocity, final velocity, acceleration, and time. For free fall, these equations are essential for calculating the distance an object travels.
Basic Kinematic Equations for Free Fall
-
Equation for Distance:
- ( d = v_0t + frac{1}{2}gt^2 )
- Where:
- ( d ) is the distance the object falls
- ( v_0 ) is the initial velocity (if the object is dropped, ( v_0 = 0 ))
- ( g ) is the acceleration due to gravity (9.8 m/s²)
- ( t ) is the time the object falls
-
Equation for Final Velocity:
- ( v = v_0 + gt )
- Where:
- ( v ) is the final velocity of the object after falling for time ( t )
-
Equation Relating Velocity, Acceleration, and Distance:
- ( v^2 = v_0^2 + 2gd )
- This equation is particularly useful when time is not known but the initial and final velocities, as well as the distance, are known.
Impact of Acceleration on Free Fall Distance
The acceleration due to gravity directly influences the distance an object covers during free fall. The kinematic equations show that the distance is proportional to the square of the time multiplied by the acceleration. This means that as time increases, the distance covered increases exponentially, and the acceleration due to gravity scales this increase.
- Example: If an object is dropped (initial velocity is zero) from a height and falls for ( t ) seconds, the distance it falls is calculated as ( d = frac{1}{2}gt^2 ). If ( t = 1 ) second, then ( d = frac{1}{2} times 9.8 times 1^2 = 4.9 ) meters. If ( t = 2 ) seconds, then ( d = frac{1}{2} times 9.8 times 2^2 = 19.6 ) meters. This demonstrates how the distance increases significantly with time due to constant acceleration.
Practical Applications
Understanding the relationship between acceleration and free fall distance has several practical applications:
- Engineering: Engineers use these principles to design structures and safety systems, such as calculating the impact force of falling objects on buildings or designing amusement park rides.
- Sports: Athletes and coaches use these concepts to improve performance in sports like diving, skydiving, and gymnastics.
- Accident Analysis: Accident investigators use kinematic equations to reconstruct events and determine the factors that contributed to accidents, such as falls from heights.
Example Calculation
Let’s calculate the distance an object falls in 3 seconds, starting from rest (initial velocity is 0 m/s).
- Given:
- ( g = 9.8 , text{m/s}^2 )
- ( t = 3 , text{s} )
- ( v_0 = 0 , text{m/s} )
- Using the formula:
- ( d = v_0t + frac{1}{2}gt^2 )
- ( d = (0 times 3) + frac{1}{2} times 9.8 times 3^2 )
- ( d = 0 + frac{1}{2} times 9.8 times 9 )
- ( d = 4.9 times 9 )
- ( d = 44.1 , text{meters} )
Thus, an object falls 44.1 meters in 3 seconds when starting from rest, due to the constant acceleration of gravity.
4. What Is Terminal Velocity And How Does It Affect Free Fall Distance?
Terminal velocity is the maximum speed an object reaches during free fall, occurring when air resistance equals the gravitational force. This significantly affects the distance an object can travel, especially over longer falls, by limiting its acceleration.
Terminal velocity is the constant speed that a freely falling object eventually reaches when the force of air resistance equals the force of gravity. At this point, the net force on the object is zero, and it stops accelerating. Understanding terminal velocity is crucial for predicting the behavior of falling objects in real-world conditions.
Factors Affecting Terminal Velocity
Several factors influence the terminal velocity of an object:
- Object’s Weight: Heavier objects tend to have higher terminal velocities because a greater force of air resistance is required to balance the force of gravity.
- Object’s Shape and Size: The shape and size of an object significantly affect the amount of air resistance it encounters. A larger object with a greater surface area experiences more air resistance, reducing its terminal velocity. Streamlined shapes experience less resistance and thus have higher terminal velocities.
- Air Density: Air density varies with altitude and temperature. At higher altitudes, the air is less dense, resulting in lower air resistance and higher terminal velocities compared to lower altitudes.
How Terminal Velocity is Reached
When an object begins to fall, it accelerates due to gravity. As its speed increases, the force of air resistance also increases. The air resistance force opposes the gravitational force, reducing the net force and, consequently, the acceleration. Eventually, the air resistance force becomes equal in magnitude to the gravitational force, resulting in a net force of zero. At this point, the object stops accelerating and falls at a constant speed—the terminal velocity.
Formula for Terminal Velocity
The terminal velocity ( v_t ) can be estimated using the following formula:
[ v_t = sqrt{frac{2mg}{rho A C_d}} ]
Where:
- ( m ) is the mass of the object,
- ( g ) is the acceleration due to gravity (9.8 m/s²),
- ( rho ) is the density of the air,
- ( A ) is the projected area of the object (the area facing the direction of motion),
- ( C_d ) is the drag coefficient, a dimensionless number that depends on the object’s shape.
Impact on Free Fall Distance
Terminal velocity limits the maximum speed an object can reach during free fall, which in turn affects the total distance the object covers over time. Here’s how:
- Initial Acceleration Phase: Initially, the object accelerates rapidly since air resistance is minimal. During this phase, the distance covered increases exponentially with time.
- Transition Phase: As the object’s speed increases, air resistance starts to play a significant role, reducing the acceleration.
- Constant Velocity Phase: Once terminal velocity is reached, the object falls at a constant speed. The distance covered now increases linearly with time, rather than exponentially.
Examples of Terminal Velocity
- Skydiver: A skydiver without a parachute typically reaches a terminal velocity of about 55 to 60 meters per second (approximately 200 km/h or 120 mph). With an open parachute, the terminal velocity is reduced to about 5 meters per second (approximately 18 km/h or 11 mph), making for a safe landing.
- Raindrops: Small raindrops reach terminal velocities of about 8 to 10 meters per second, which is why they don’t cause significant impact when they hit the ground. Larger raindrops have higher terminal velocities.
- Feather: A feather has a very low terminal velocity due to its large surface area and low weight, causing it to float and drift slowly through the air.
Practical Implications
Understanding terminal velocity is crucial in various fields:
- Aviation: Pilots and aircraft designers consider terminal velocity when designing aircraft and parachutes to ensure safety and efficiency.
- Sports: Athletes in sports like skydiving and BASE jumping manipulate their body position to control their terminal velocity.
- Engineering: Engineers consider terminal velocity when designing structures to withstand wind loads and when analyzing the behavior of falling objects in industrial settings.
5. Can The Shape Of A Body Affect The Distance It Travels In Free Fall?
Yes, the shape of a body significantly affects the distance it travels in free fall. This is primarily due to how the shape influences air resistance, a crucial factor determining the terminal velocity and overall motion of a falling object.
The shape of a body plays a crucial role in determining how far it travels during free fall, primarily because it significantly affects the amount of air resistance the body encounters. Air resistance, also known as drag, is a force that opposes the motion of an object through the air. Here’s a detailed explanation of how shape impacts free fall distance:
How Shape Affects Air Resistance
- Surface Area: The larger the surface area of an object exposed to the air, the greater the air resistance it will experience. A flat, broad object will encounter more air resistance than a streamlined object of the same mass.
- Streamlining: Streamlined shapes are designed to minimize air resistance. These shapes allow air to flow smoothly around the object, reducing turbulence and pressure drag. Examples include teardrop shapes or the design of airplane wings.
- Drag Coefficient ((C_d)): The drag coefficient is a dimensionless number that quantifies how much an object resists motion through a fluid, such as air. It depends on the object’s shape and surface texture. A lower drag coefficient indicates less air resistance.
Impact on Terminal Velocity
The shape of an object significantly influences its terminal velocity, which is the constant speed that a freely falling object eventually reaches when the force of air resistance equals the force of gravity.
- Higher Air Resistance: Objects with shapes that create high air resistance reach lower terminal velocities. This is because the air resistance force quickly balances the gravitational force, preventing the object from accelerating further.
- Lower Air Resistance: Streamlined objects with shapes that minimize air resistance can achieve much higher terminal velocities. The air resistance takes longer to balance the gravitational force, allowing the object to accelerate to greater speeds before reaching terminal velocity.
Examples of Shape Impact
- Feather vs. Stone: A feather has a large surface area relative to its weight and an irregular shape, resulting in high air resistance and a low terminal velocity. This causes it to float and drift slowly through the air. A stone, on the other hand, has a smaller surface area and a more compact shape, resulting in lower air resistance and a much higher terminal velocity, causing it to fall quickly.
- Skydiver Positions: Skydivers manipulate their body position to control their shape and, consequently, their air resistance and terminal velocity. By spreading out their limbs and body, they increase their surface area and air resistance, reducing their terminal velocity. By streamlining their body, they decrease air resistance and increase their terminal velocity.
- Parachutes: Parachutes are designed to have a large surface area, which creates high air resistance and drastically reduces the terminal velocity of a falling person, allowing for a safe landing.
Mathematical Representation
The force of air resistance ((F_d)) can be described by the following equation:
[ F_d = frac{1}{2} rho v^2 C_d A ]
Where:
- (rho) is the air density,
- (v) is the velocity of the object,
- (C_d) is the drag coefficient (dependent on shape),
- (A) is the projected area.
This equation shows that the force of air resistance is directly proportional to the drag coefficient ((C_d)) and the projected area ((A)), both of which are heavily influenced by the object’s shape.
Practical Implications
Understanding how the shape of a body affects its free fall distance has several practical applications:
- Aerospace Engineering: Aircraft and spacecraft are designed with streamlined shapes to minimize air resistance and improve fuel efficiency.
- Automotive Engineering: Cars are designed with aerodynamic shapes to reduce drag and improve performance and fuel economy.
- Sports Equipment: Equipment used in sports like skiing, cycling, and swimming are designed to minimize air or water resistance and improve athletic performance.
6. How Does Air Resistance Affect The Distance Traveled During Free Fall?
Air resistance significantly affects the distance traveled during free fall by opposing the motion and reducing acceleration. It leads to the concept of terminal velocity, limiting the maximum speed and thus the distance covered over time.
Air resistance, also known as drag, is a force that opposes the motion of an object through the air. It plays a crucial role in determining how far an object travels during free fall. Here’s a detailed explanation of how air resistance affects the distance traveled:
Basic Principles of Air Resistance
-
Definition: Air resistance is the force exerted by air on a moving object, acting in the opposite direction to the object’s motion.
-
Factors Affecting Air Resistance:
- Speed: Air resistance increases with the speed of the object. The faster the object moves, the greater the air resistance.
- Shape and Size: The shape and size of the object affect the amount of air resistance it encounters. Objects with larger surface areas experience more air resistance.
- Air Density: Air density also affects air resistance. Higher density air exerts a greater force on the object.
Mathematical Representation
The force of air resistance ((F_d)) can be described by the following equation:
[ F_d = frac{1}{2} rho v^2 C_d A ]
Where:
- (rho) is the air density,
- (v) is the velocity of the object,
- (C_d) is the drag coefficient (dependent on the object’s shape),
- (A) is the projected area of the object.
Impact on Acceleration
- Initial Phase: When an object starts to fall, the only force acting on it is gravity ((F_g = mg), where (m) is mass and (g) is the acceleration due to gravity). The object accelerates downwards.
- Increasing Speed: As the object’s speed increases, air resistance ((Fd)) also increases. The net force on the object is now the difference between the gravitational force and the air resistance: (F{net} = F_g – F_d).
- Reduced Acceleration: Because air resistance opposes gravity, the net force is reduced, leading to a decrease in the object’s acceleration. The object continues to accelerate, but at a slower rate.
Terminal Velocity
- Definition: Terminal velocity is the constant speed that a freely falling object eventually reaches when the force of air resistance equals the force of gravity. At this point, the net force on the object is zero, and it stops accelerating.
- Reaching Terminal Velocity: As the object falls, air resistance increases until it equals the gravitational force ((F_d = F_g)). At this point, the net force is zero, and the object no longer accelerates. It falls at a constant speed—the terminal velocity.
Impact on Distance Traveled
- Early Stages of Fall: In the early stages of the fall, when the object is accelerating, the distance it travels increases rapidly with time. The effect of air resistance is minimal during this phase.
- Later Stages of Fall: As the object approaches terminal velocity, the rate at which it covers distance changes. The distance covered increases linearly with time because the object is no longer accelerating but moving at a constant speed.
Comparison to Free Fall in a Vacuum
In a vacuum, there is no air resistance, and an object would continue to accelerate indefinitely due to gravity. The distance it travels would increase quadratically with time, as described by the equation:
[ d = frac{1}{2} g t^2 ]
Where (d) is distance, (g) is the acceleration due to gravity, and (t) is time.
In contrast, in the presence of air resistance, the object’s acceleration decreases, and it eventually reaches terminal velocity, limiting the maximum speed and distance covered over time.
Practical Examples
- Skydiver: A skydiver initially accelerates downwards. As their speed increases, air resistance also increases, eventually reaching a point where it equals the force of gravity. At this point, the skydiver reaches terminal velocity.
- Feather vs. Stone: A feather experiences significant air resistance due to its shape and low weight, resulting in a low terminal velocity and a slow descent. A stone, with its smaller surface area and higher weight, experiences less air resistance and reaches a higher terminal velocity, resulting in a faster descent.
Summary Table
| Factor | Effect on Free Fall |
|---|---|
| Air Resistance | Opposes motion, reduces acceleration. |
| Speed | Higher speed leads to greater air resistance. |
| Shape and Size | Larger surface area increases air resistance. |
| Air Density | Higher air density increases air resistance. |
| Terminal Velocity | Limits the maximum speed of the falling object, causing the distance covered to increase linearly with time once reached, rather than exponentially. |
| Vacuum | No air resistance, object accelerates continuously, and distance increases quadratically with time. |
7. How Does Altitude Affect Free Fall Distance?
Altitude affects free fall distance primarily by influencing air density and gravitational acceleration. Higher altitudes have lower air density, which reduces air resistance, and slightly weaker gravitational pull, impacting both terminal velocity and acceleration.
Altitude influences the distance an object travels in free fall through two primary factors: air density and gravitational acceleration. Here’s a detailed look at how each of these affects free fall.
Air Density
-
Definition: Air density refers to the mass of air per unit volume. It decreases with increasing altitude because there is less air pressing down from above.
-
Impact on Air Resistance: Air resistance, also known as drag, is the force that opposes the motion of an object through the air. It is directly proportional to air density. Therefore, as altitude increases and air density decreases, air resistance decreases.
-
Mathematical Representation: The force of air resistance ((F_d)) is given by:
[ F_d = frac{1}{2} rho v^2 C_d A ]
Where:
- (rho) is the air density,
- (v) is the velocity of the object,
- (C_d) is the drag coefficient (dependent on the object’s shape),
- (A) is the projected area of the object.
-
Effect on Terminal Velocity: Because air resistance decreases with altitude, an object falling from a higher altitude will experience less drag, allowing it to accelerate for a longer period before reaching its terminal velocity. This results in a higher terminal velocity at higher altitudes compared to lower altitudes.
Gravitational Acceleration
-
Definition: Gravitational acceleration ((g)) is the acceleration experienced by an object due to the force of gravity. While often considered constant at 9.8 m/s², it actually varies slightly with altitude.
-
Impact on Gravity: Gravitational acceleration decreases with increasing distance from the Earth’s center. Since altitude represents an increase in this distance, gravitational acceleration is slightly weaker at higher altitudes.
-
Mathematical Representation: The gravitational force ((F_g)) is given by:
[ F_g = frac{GMm}{r^2} ]
Where:
- (G) is the gravitational constant ((6.674 times 10^{-11} , text{N}cdottext{m}^2/text{kg}^2)),
- (M) is the mass of the Earth,
- (m) is the mass of the object,
- (r) is the distance from the center of the Earth to the object.
As (r) increases (higher altitude), (F_g) and, consequently, (g) decrease.
-
Effect on Free Fall Distance: The decrease in gravitational acceleration at higher altitudes means that the object accelerates slightly less than it would at lower altitudes. This effect is generally small but can become significant over very large distances.
Combined Effects
-
Initial Phase of Fall:
- At higher altitudes, the object experiences less air resistance and slightly lower gravitational acceleration. The reduced air resistance allows the object to accelerate more freely than at lower altitudes.
-
Reaching Terminal Velocity:
- Because air density decreases with altitude, the object will reach a higher terminal velocity compared to falling from a lower altitude.
-
Overall Distance:
- For a given time interval, an object falling from a higher altitude will generally cover a greater distance than one falling from a lower altitude due to the combined effects of reduced air resistance and a slightly higher terminal velocity.
Quantitative Example
Let’s consider two scenarios:
- Low Altitude: An object falling from 1000 meters above sea level.
- High Altitude: An object falling from 5000 meters above sea level.
In the high-altitude scenario, the object will experience:
- Lower air density, leading to reduced air resistance.
- Slightly weaker gravitational acceleration.
The object at 5000 meters will likely reach a higher terminal velocity and cover a greater distance in the same amount of time compared to the object at 1000 meters.
Practical Implications
- Skydiving: Skydivers need to account for altitude when planning their jumps. The higher the altitude, the longer they will accelerate and the faster they will fall before reaching terminal velocity.
- Aviation: Aircraft performance is affected by altitude due to changes in air density. Higher altitudes can result in reduced drag but also require adjustments to engine power and lift.
- Meteorology: Understanding how altitude affects the motion of objects in the atmosphere is crucial for predicting weather patterns and the behavior of falling objects like hailstones.
Summary Table
| Factor | Effect at Higher Altitudes |
|---|---|
| Air Density | Decreases, leading to reduced air resistance. |
| Gravitational Acceleration | Slightly decreases, leading to a slightly lower force of gravity. |
| Terminal Velocity | Increases, as reduced air resistance allows the object to accelerate to higher speeds. |
| Overall Distance | Generally greater for a given time interval due to reduced air resistance and a slightly higher terminal velocity, although the effect of decreased gravitational acceleration is comparatively minimal. |
8. How Do Initial Conditions Affect Free Fall Distance?
Initial conditions, such as initial velocity and height, significantly affect the distance an object travels in free fall. Initial velocity adds to or subtracts from the distance covered, while initial height determines the total potential distance of the fall.
The initial conditions under which an object begins its free fall significantly influence the distance it will travel. These conditions primarily include initial velocity and initial height. Let’s explore how each of these affects the free fall distance.
Initial Velocity
-
Definition: Initial velocity ((v_0)) is the velocity of the object at the moment it begins its free fall. This can be zero if the object is simply dropped, or it can have a non-zero value if the object is thrown or projected downwards or upwards.
-
Impact on Downward Initial Velocity: If the object is thrown downwards, the initial velocity adds to the distance covered during the fall. The kinematic equation that describes this situation is:
[ d = v_0t + frac{1}{2}gt^2 ]
Where:
- (d) is the distance traveled,
- (v_0) is the initial downward velocity,
- (t) is the time of the fall,
- (g) is the acceleration due to gravity.
In this case, the total distance fallen will be greater compared to an object dropped from rest ((v_0 = 0)).
-
Impact on Upward Initial Velocity: If the object is thrown upwards, the initial velocity opposes the force of gravity. The object will initially move upwards, decelerating until it reaches its highest point, where its velocity momentarily becomes zero. Then, it will start to fall back down. The kinematic equations to describe this situation are more complex but can be broken down into two phases:
- Upward Motion:
- (v = v_0 – gt) (velocity at time (t))
- (d = v_0t – frac{1}{2}gt^2) (distance above the starting point at time (t))
- The time to reach the highest point ((t{up})) can be found when (v = 0): (t{up} = frac{v_0}{g})
- The maximum height reached ((d{max})) is: (d{max} = frac{v_0^2}{2g})
- Downward Motion:
- The object falls from the maximum height ((d_{max})) with zero initial velocity.
- The time to fall back to the starting point ((t{down})) is the same as (t{up}).
- The total distance traveled from the start to the return to the start is zero (displacement).
- Upward Motion:
Initial Height
-
Definition: Initial height ((h_0)) is the starting height of the object above a reference point, usually the ground.
-
Impact on Potential Distance: The initial height determines the total potential distance the object can fall. The higher the initial height, the greater the potential distance. The total time the object spends in free fall depends on this initial height.
-
Kinematic Equation: The equation to find the time (t) it takes for an object to fall from an initial height (h_0) to the ground (assuming no initial velocity) is derived from:
[ h_0 = frac{1}{2}gt^2 ]
Solving for (t):
[ t = sqrt{frac{2h_0}{g}} ]
The total distance fallen is equal to the initial height (h_0).
Combined Effects
-
Object Dropped from a Height:
- If an object is simply dropped ((v_0 = 0)) from a height (h_0), the distance it falls is (h_0), and the time it takes to fall is determined by (t = sqrt{frac{2h_0}{g}}).
-
Object Thrown Downwards from a Height:
-
If an object is thrown downwards with an initial velocity (v_0) from a height (h_0), the distance it falls is still effectively (h_0), but the time it takes to fall is reduced because of the additional initial velocity. The equation becomes more complex:
[ h_0 = v_0t + frac{1}{2}gt^2 ]
This quadratic equation can be solved for (t).
-
-
Object Thrown Upwards from a Height:
- If an object is thrown upwards with an initial velocity (v_0) from a height (h_0), it will first travel upwards, reach a maximum height, and then fall back down. The total distance fallen will be the maximum height reached plus the initial height, and the time to fall will be the sum of the time to reach the maximum height and the time to fall back to the ground.
Practical Examples
- Skydiving: The initial height of the skydiver when jumping from the plane determines the total time they have in free fall. Their initial velocity (usually zero relative to the plane) and any maneuvers they perform during the fall will affect their trajectory and the distance covered horizontally.
- Dropping a Ball from a Building: The height of the building determines the potential distance the ball can fall. If the ball is thrown downwards, it will reach the ground faster than if it is simply dropped.
- Throwing a Ball Upwards: The initial velocity of the ball thrown upwards determines how high it will go and how long it will take to return to the ground.
Summary Table
| Initial Condition | Impact on Free Fall |
|---|---|
| Initial Velocity | Downward velocity increases the distance covered in a given time. Upward velocity reduces the immediate distance and affects the trajectory, requiring more complex calculations. |
| Initial Height | Determines the total potential distance the object can fall and affects the total time the object spends in free fall. |
9. How Do Different Gravitational Fields Affect Free Fall Distance?
Different gravitational fields, such as those on the Moon versus Earth, drastically alter the acceleration and thus the distance an object travels in free fall. Lower gravity means slower acceleration and greater hang time for the same drop height.
The gravitational field in which an object is located significantly impacts the distance it travels during free fall. The strength of the gravitational field determines the acceleration of the object, which in turn affects the distance covered over a given period. Let’s explore how different gravitational fields affect free fall distance.
Basic Principles of Gravitational Fields
- Definition: A gravitational field is the region around a massive object in which other objects experience a force due to gravity. The strength of the gravitational field is determined by the mass of the object creating the field and the distance from that object.
- Gravitational Acceleration (g): Gravitational acceleration is the acceleration experienced by an object due to the force of gravity. It is denoted by (g) and varies depending on the mass of the celestial body and the distance from its center. On Earth, (g) is approximately (9.8 , text{m/s}^2).
- **Newton’



